Forensic Mathematics: A Newer Approach to Forensics and Solving Crime

The following post is authored by Shreyas Patel. The post depicts recent developments and the need for Forensic Mathematics along with real problem-solving examples.

Introduction to Forensic Mathematics

When we used to study trigonometry, statistics, and probability— as a part of Statistics— in Mathematics, we used to wonder with questions like what is the need to study them at all? Are we ever going to use it in our day-to-day life?

Many people still don’t know the importance of these terms in mathematics.

But what if I ask you, “Is it possible to solve crime with the help of Mathematics?“

Did you ever think about it in this way? If yes, then do you know in which way mathematics is used to solve crimes and help forensics?

Mathematics is one of the most important techniques used in any forensic discipline to solve problems related to uncertainty, probabilities, and interpretation of results which may be indirectly helpful to come up with a predictable solution.

So, according to its applications,

Forensic Mathematics is defined as an application of the principles and methods of mathematics such as trigonometry, probability, statistics, graphical representation & many more for the determination of various mathematical quantities which are considered to be a part of the different methods/tests used to analyze the evidence related to a crime in order to serve justice on behalf of the Criminal Justice System.

It can also be said that Forensic Mathematics is an application of mathematical concepts in the field of forensic science with the purpose of solving crimes.

When statistics are used as an application to forensic sciences, it is called Forensic Statistics. It can also be considered as the subfield of forensic mathematics.

Also Read:

• Most Famous Forensic Anthropologist With Their Achievements and Discoveries
• How to Prepare for NTA UGC NET/JRF Forensic Science Paper 2?

Need of Mathematics in Forensic Science 

In Forensic Science, it won’t be possible to analyze any evidence without applying Mathematics.

Investigating Officers/ Experts/ Analysts have to measure the dimensions of evidence, establish the height from which a blood drop would have originated, or establish/define the boundary/area of a crime scene which constitutes a few applications of Mathematics.

Different branches of forensic science such as forensic biology, forensic chemistry, forensic physics, forensic ballistics, etc. incorporate the concepts and principles of mathematics to analyze and interpret the observations and results in order to be able to present the findings with a significant mathematical value to support it.

Applications of Mathematics in Forensic Science

In forensic anthropology, a forensic anthropologist would measure the dimensions of different types of bones and classify them on the basis of:

• Different Racial groups,
• Determination of sex, and
• Approximate age estimation of an individual through skeletal remains.

All these classifying parameters use different indices such as Cephalic Index, Sternal Index, Ischiopubic index, etc. to give a possible interpretation of skeletal remains. This is one of the many examples of the incorporation of mathematics in the field of forensic science. [5]

A. Trigonometry Measurements:

Trigonometry is the application of mathematics for solving problems involving the estimation of angles using different trigonometric functions such as sine, cosine, tangent, and their inverse functions such as sec, cosec, and cot.

Majorly, trigonometry is as useful in forensics as it is in the field of engineering.

Trigonometry holds special importance in the hearts of Forensic Experts. Trigonometry plays a vital role in the field of forensic science, especially in Bloodstain Pattern Analysis with respect to the problems related to the estimation of the height of the source of blood & distance of the bloodstains and Forensic Ballistics.

Trigonometric methods enable the bloodstain analyst to gain an understanding of the angles at which the objects (weapons) have impacted the source of blood on the basis of the measurement of a bloodstain which is significant for Crime Scene Reconstruction. [1]

B. Statistical Interpretation

Statistical interpretation also has an important role in the field of forensic science. For example, if you make repetitive diametric measurements of a single fiber or of a set of fibers from the same fabric or cloth, they won’t be identical if measured with sufficiently high accuracy.

Likewise, repetitive measurements of the Refractive Index or Density of glass fragments will have slightly varying values. At the time of interpretation of the observations, it is necessary to quantify such variations in measurements or take them into consideration when comparing results from different sources/samples.

Therefore, a forensic scientist must be able to determine the magnitude and significance of the variability and uncertainty in his/her work. [2]

C. Probability of Uncertainty

In any forensic science investigation, the investigating officer needs to deal with the uncertainty of the data.

There are different types of probability measurements used in forensic science. Evidence collected from a crime scene may need to be compared with a reference or suspected materials/samples. [2]

Example: If you have 1 questioned handwriting sample which belongs to a Left-Handed person and there are a total of 10 suspect samples out of which 7 are Right-Handed and 3 are Left-Handed.

In this case, probability measurements can be incorporated to determine the possibility of the questioned handwriting sample belonging to one of those suspect samples.

One of the most useful types of probability is conditional probability. Conditional probabilities are particularly useful in the forensic discipline because they form the basis of Bayes’ theorem and using Bayes’ theorem (Bayesian statistics) forensic scientists can work with conditional probabilities. [1]

Also Read:

• Adulteration of Cement: Test, Chemical & Instrumental Analysis
• Age Estimation From Teeth: All Forensic Odontology Tables Included

Solving Problems Related To Forensics Using Mathematics

A. Trigonometry Examples

Problem 1: Forensic Ballistics

One person from the audience claims that he has been hit by a cricket ball thrown by the suspect. Test results show that the initial speed could be a maximum of 50 m/s and not more than it.  If the suspect was 150 m away from that person, determine whether that person was within the range or not.

Solution :

Here, We can calculate the maximum range when the initial speed is 50 m/s.

In projectile motion, the formula of range is ^[3]

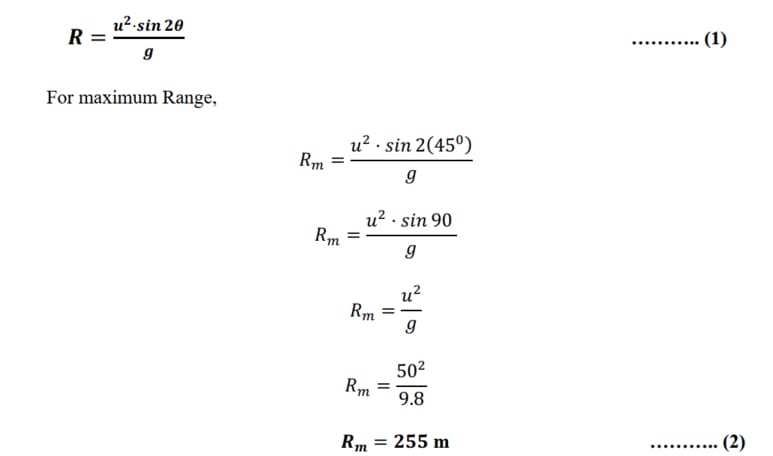
So, if the initial speed is 50 m/s then the Maximum Range would be 255 m.

Let’s calculate the initial speed when max. the range is 150 m.

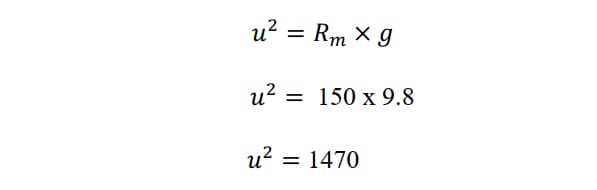
    u =  38.34 m/s

So, if the audience is at 150 m then the initial speed would be 38.34 m/s.

So, we can say that the person is in range.

Problem 2: Blood Stain Analysis

Bloodstains of width 5.5 mm and a length of 7 mm are identified from an injured person, who was running away at 2 m/s speed from a crime scene. As an investigation officer, determine whether you will consider that this bloodstain arises from a leg or hand wound. Consider the injured man is enough tall.

Solution :

First Calculate the impact angle, ^[4]

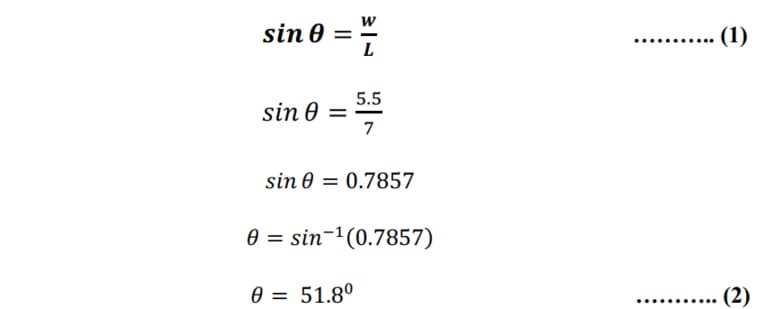
So, the impact angle is 51.80°

For moving sources,

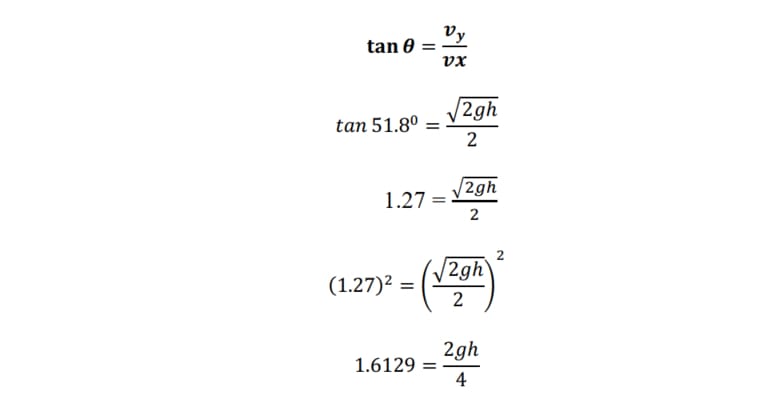
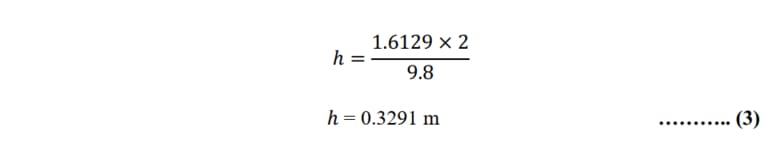
The Height of the wound is 0.3291 m. So, the Investigation officer can say that the bloodstain arises from a leg.

B. Probability Examples

Problem 1: Blood Grouping

A bag has been stolen from a desk drawer in the office where there are 30 people are present. Assume that, it is confirmed that someone within the office has stolen the bag. While stealing the bag, the thief got injured and a drop of blood fell on a desk drawer. A blood drop on the desk drawer is found to be group A; There are five people with A blood group in that office. Calculate the probability of selecting someone at random who is guilty and has an A blood group.

Solution :

Only one person is guilty:  P (G) = 1 / 30
The guilty person has A blood group : P (A|G) = 1 
Probability of finding group A in the office: P (B) = 5 / 30 
For those with group A blood, one is guilty: P (G|A) = 1 / 5

The probability of selecting someone at random who is guilty and has A blood group = 1 / 5

Problem 2: Hair Analysis

The Investigation Officer founds 15 hair samples in one bedroom. He selects 8 hairs for analysis from 15. Calculate how many combinations of these hairs are possible in the sample.

Solution :

There is a formula available for evaluating the number of combinations that are possible when selecting m items from a total of n. ^[1]

ⁿ C _m   =   n !/ m! (n − m) !

 ¹⁵ C ₈   =  15 ! /8! (15 − 8) !

 ¹⁵ C ₈   =  (15 x 14 x 13x 12 x 11 x 10 x 9 x 8!)/  (8! X 7!)

¹⁵ C ₈   =  (15 x 14 x 13x 12 x 11 x 10 x 9 )/ (7 x 6 x 5 x 4 x 3 x 2 x 1) 

 ¹⁵ C ₈   =       6435   

So, there is a total of 6435 combinations of hairs are possible in his sample.

Conclusion

From all the above practical examples, it can be concluded that mathematics is a subject/discipline which can be incorporated in all types of sciences/scientific disciplines including the various branches of forensic science such as forensic biology, forensic chemistry, forensic physics, forensic ballistics, etc.

Mathematics has a vast scope in forensic science because it deals with the analysis of the evidence followed by its interpretation, corroborating it with mathematical values/calculations.

In forensic science, scientists have to explain how likely is it that their observations/findings are accurate which could be determined through probability, so, mathematics actually backs up the data.

Moreover, only with the mathematic interpretation, it is possible to determine the findings such as the height from which a blood drop has originated (the location where a source of blood received a blow/an impact), or the angle at which a blood drop has struck a target surface resulting in the formation of bloodstains, or the probability that the blood types of any two randomly chosen individuals would match with each other and much more.

As it is clearly evident that mathematical calculations have a wide range of applications in forensic science. So, for a forensic expert, it is vital to possess knowledge of mathematics along with the principles and theories of different branches and disciplines of forensic science to solve a crime efficiently and effectively.

References :

1. Adam C. Essential Mathematics and Statistics for Forensic Science. John Wiley & Sons; 2011 Sep 20 [Link]
2. Lucy D. Introduction to Statistics for Forensic Scientists. John Wiley & Sons; 2013 May 3.
3.  Maximum Height, Time of Flight and Horizontal Range of a Projectile | Formula, Definition, Diagrams [Link]  
4. James SH, Kish PE, Sutton TP. Principles of Bloodstain Pattern Analysis: Theory and Practice. CRC Press; 2005 May 26.
5. Dr. K.S. Narayan Reddy, Dr. O.P. Murty. The Essentials of Forensic Medicine and Toxicology. Jaypee Brothers Medical Publishers (P) Ltd. 33^rd Edition; 2014.